scivilla.blogg.se - Quadratic programming

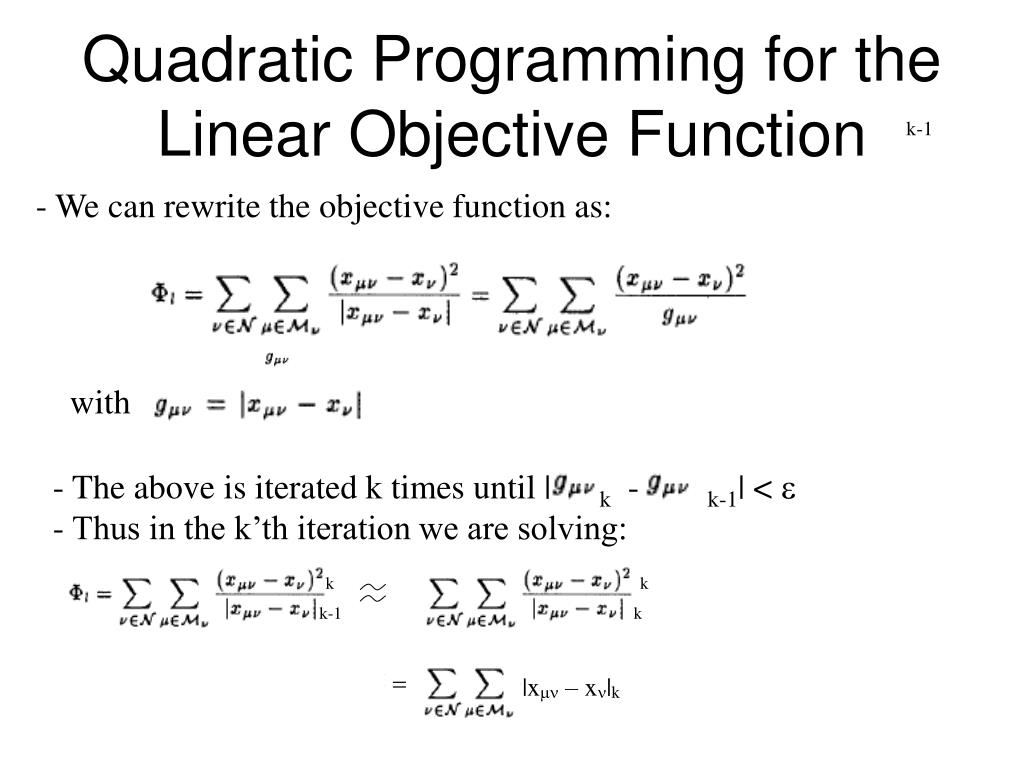
The author would like to express his sincere thanks to the anonymous referees and editors for insightful comments and useful suggestions. Nguyen Nang Tam for valuable suggestions. Nguyen Dong Yen for comments that greatly improved the paper. Questions on quadratic programming, the optimization of a quadratic objective function subject to affine constraints.

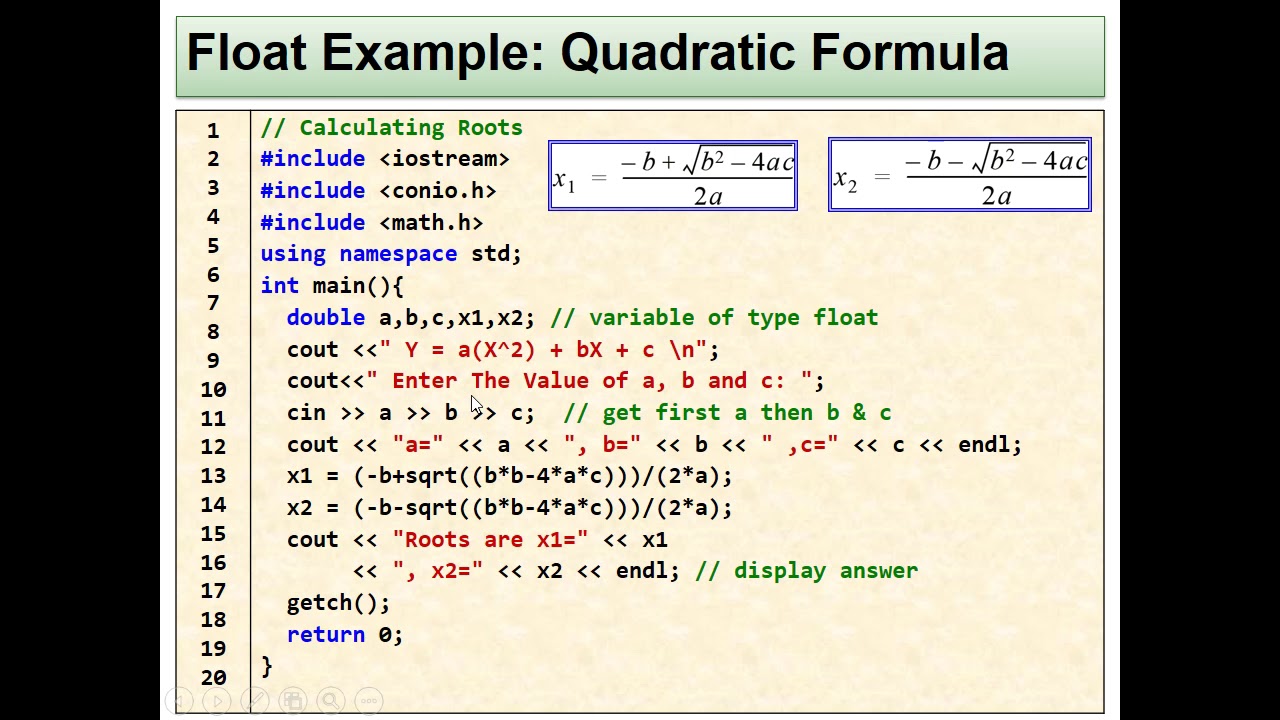
Its general form is minimize f(x) : 1 2 xTBx xTb (3.1a) over x 2 lRn subject. Such an NLP is called a Quadratic Programming (QP) problem. Supported by the Hanoi University of Industry. Chapter 3 Quadratic Programming 3.1 Constrained quadratic programming problems A special case of the NLP arises when the objective functional f is quadratic and the constraints h g are linear in x 2 lRn. Science and Technology Development (NAFOSTED) under grant number 101.01-2018.306. This research is funded by Vietnam National Foundation for As special cases, we obtain optimality conditions for the quadratic programming problems under linear constraints in Hilbert spaces. This means that if there is a solution to the primal minimization problem, then there is a solution to the dual maximization problem, and the dual maximum value is equal to the primal minimum value.In this paper, we give optimality conditions for the quadratic programming problems with constraints defined by finitely many convex quadratic constraints in Hilbert spaces. For quadratic optimization, strong duality holds if is positive semidefinite.The relationship between the factored dual vector and the unfactored dual vector is.With a factored quadratic objective, the dual problem may also be expressed as:.The Lagrangian dual problem for quadratic optimization with objective is given by: ».The dual maximizer provides information about the primal problem, including sensitivity of the minimum value to changes in the constraints.

The dual maximum value is always less than or equal to the primal minimum value, so it provides a lower bound.

The primal minimization problem has a related maximization problem that is the Lagrangian dual problem.

The objective function may be specified in the following ways:.

With QuadraticOptimization, parameter equations of the form par  val, where par is not in vars and val is numerical or an array with numerical values, may be included in the constraints to define parameters used in f or cons.

The constraints cons can be specified by:.

Vector variable restricted to the geometric region Variable with name and dimensions inferred

The variable specification vars should be a list with elements giving variables in one of the following forms:.

When the objective function is real valued, QuadraticOptimization solves problems with by internally converting to real variables, where and.

Mixed-integer quadratic optimization finds and that solve the problem:.

The space consists of symmetric positive semidefinite matrices.

Quadratic optimization finds that solves the primal problem: ».

In this sense, QPs are a generalization of LPs and a special case of the general nonlinear programming problem.

Quadratic optimization is a convex optimization problem that can be solved globally and efficiently with real, integer or complex variables. CHAPTER 2: QUADRATIC PROGRAMMING Overview Quadratic programming (QP) problems are characterized by objective functions that are quadratic in the design variables, and linear constraints.

Quadratic optimization is typically used in problems such as parameter fitting, portfolio optimization and geometric distance problems.

Quadratic optimization is also known as quadratic programming (QP) or linearly constrained quadratic optimization.